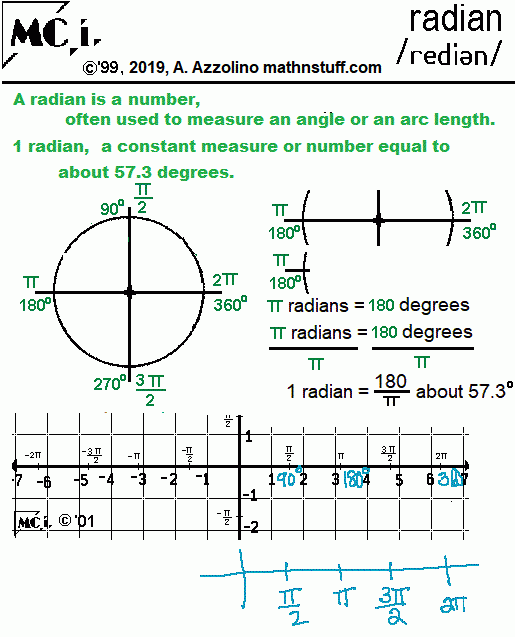
Understanding coterminal angles in both degrees and radians is an essential concept in trigonometry. Coterminal angles are angles that share the same terminal side in the coordinate plane. These angles can differ by multiples of 360 degrees or 2π radians, making them equivalent in terms of their position on the unit circle. Working with coterminal angles helps simplify calculations and problem-solving in trigonometry.
When working with coterminal angles, it is important to be able to convert between degrees and radians. This conversion allows us to express angles in different units and manipulate them easily in trigonometric functions. A coterminal angles degree and radian worksheet can provide practice problems for students to hone their skills in converting between these units and identifying coterminal angles in various scenarios.
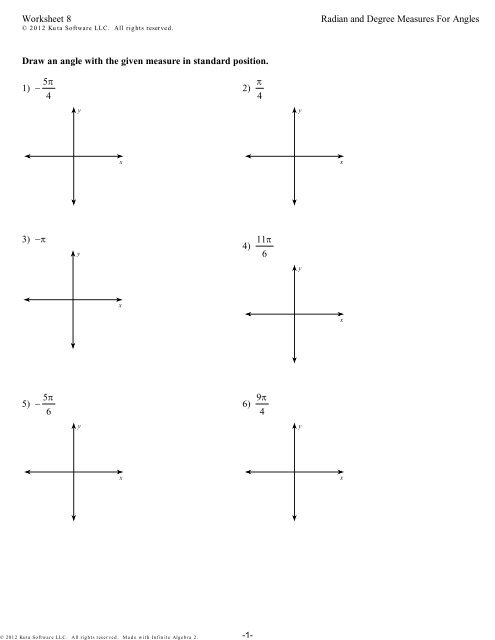 Worksheet 8 Radian And Degree Measures For Angles Draw An (www.yumpu.com)
Worksheet 8 Radian And Degree Measures For Angles Draw An (www.yumpu.com)
One common type of problem on a coterminal angles degree and radian worksheet is to find coterminal angles for a given angle, both in degrees and radians. Students may be asked to identify positive and negative coterminal angles for a given angle and express them in both units. This exercise helps reinforce the concept of coterminal angles and their relationship to the unit circle.
Another type of problem on the worksheet may involve determining the smallest positive coterminal angle for a given angle, either in degrees or radians. This requires students to understand the periodic nature of trigonometric functions and find the angle within one full revolution that is coterminal with the given angle. Practicing these problems can improve students’ proficiency in working with coterminal angles and understanding their properties.
In addition to finding coterminal angles, a coterminal angles degree and radian worksheet may also include problems involving the sum or difference of angles. Students may be asked to find the sum or difference of two coterminal angles, either in degrees or radians, and express the result in both units. This type of problem challenges students to apply their knowledge of trigonometric identities and properties of coterminal angles.
In conclusion, a coterminal angles degree and radian worksheet is a valuable tool for practicing and mastering the concept of coterminal angles in trigonometry. By working through various problems involving coterminal angles, students can improve their skills in converting between degrees and radians, identifying coterminal angles, and manipulating angles in trigonometric functions. This practice is essential for building a strong foundation in trigonometry and preparing for more advanced topics in mathematics.